Math, Sleeves and Xah in Second Life

polyhedron n nekos
A regular polyhedron structure. The polyhedron structure is createdy by Sleeves Rhode (the girl avatar above). The anime drawing is by Altair Woodget.
polyhedron n nekos 2
polyhedron n catgirl
Truchet tiles
Truchet tiles. Floor by Colin Fizgig, tube by Sleeves Rhode.

For a 3D version by Sleeves, see: Celtic Knots, Truchet tiles, Combinatorial Patterns.

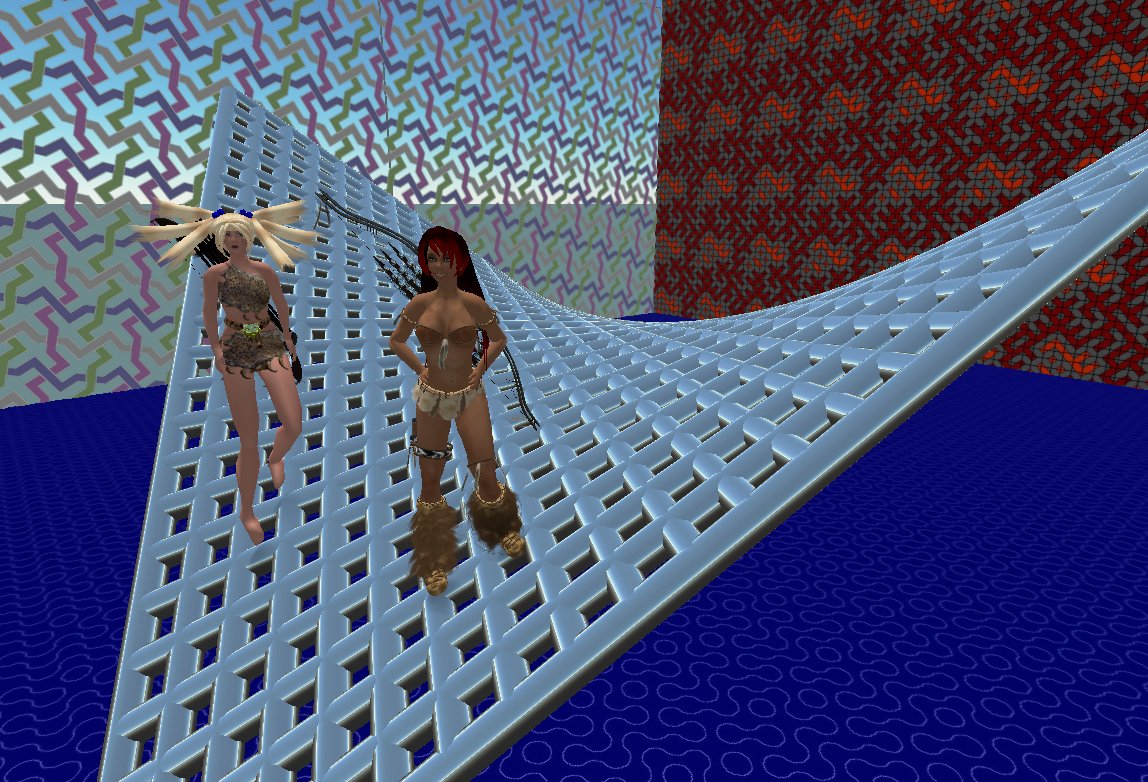
hyperbolic paraboloid
This surface is called hyperbolic-paraboloid, made my yours truely Xah Toll. It is a surface made entirely of (straight) lines. From this close up, one can see how this surface is curved, yet straight!

It is called hyperbolic-paraboloid because the horizontal cross sections are hyperbolas and diagonal vertical cross-sections are parabolas. This structure is commonly used as roofs for modern pavillions.

You can easily make a hyperbolic-paraboloid. Imagine a cube. Mark the top two opposite corners. These two corners will be the top two vertexes of the surface you see in the above image. Now, also mark the two opposite corners at the bottom of the cube. Draw diagonal lines from these corners. (look at the figure above. Your diagonal lines will be the edges of the surface) Mark regular intervals on these diagonal lines. Now, connect lines from one diagonal to the other side. You are all done!

For a Java applet that does live rotation of hyperbolic-paraboloid, see hyperbolic-paraboloid.

tiling dragon
A dragon (Capt Carroll) posting behind a tiling and symmetry exhibition. These tiles are by yours truely (Xah Toll). See Introduction to Symmetry.

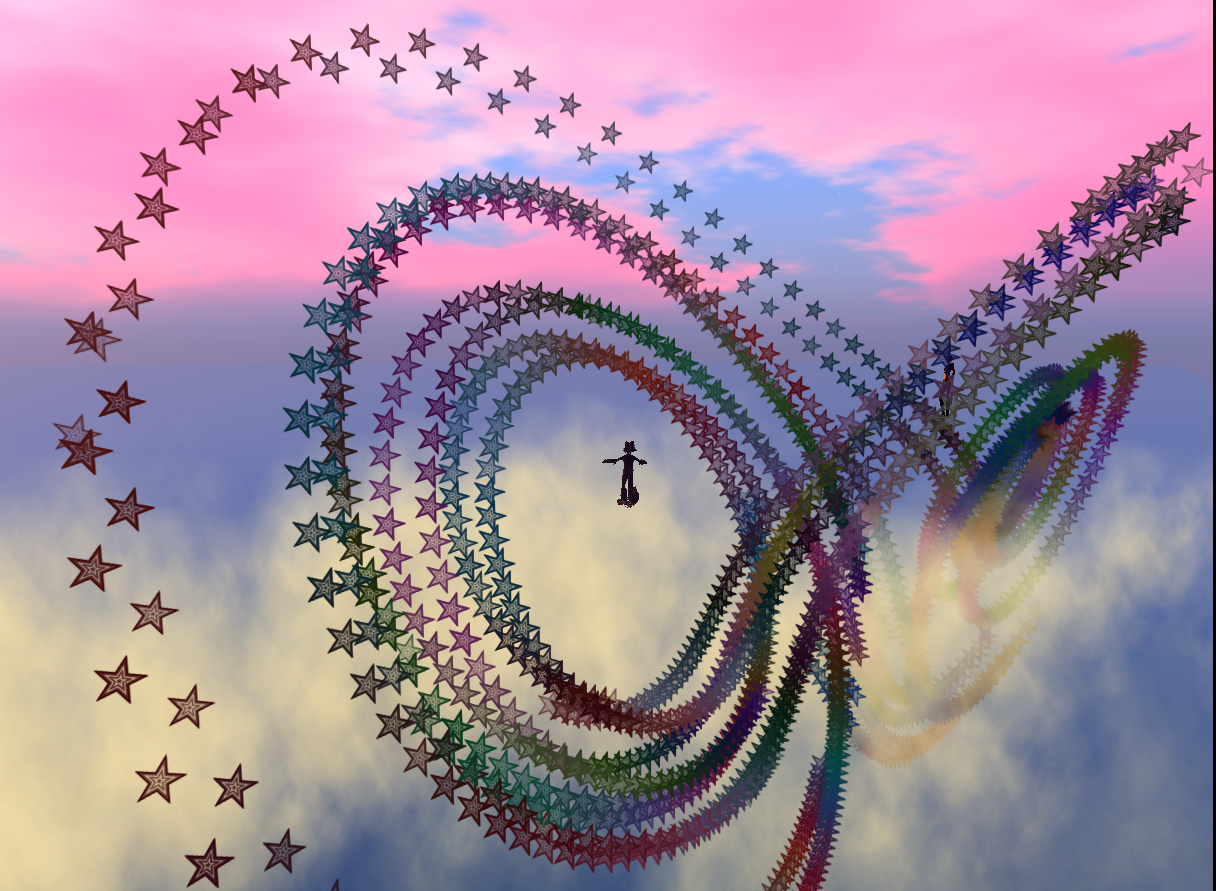
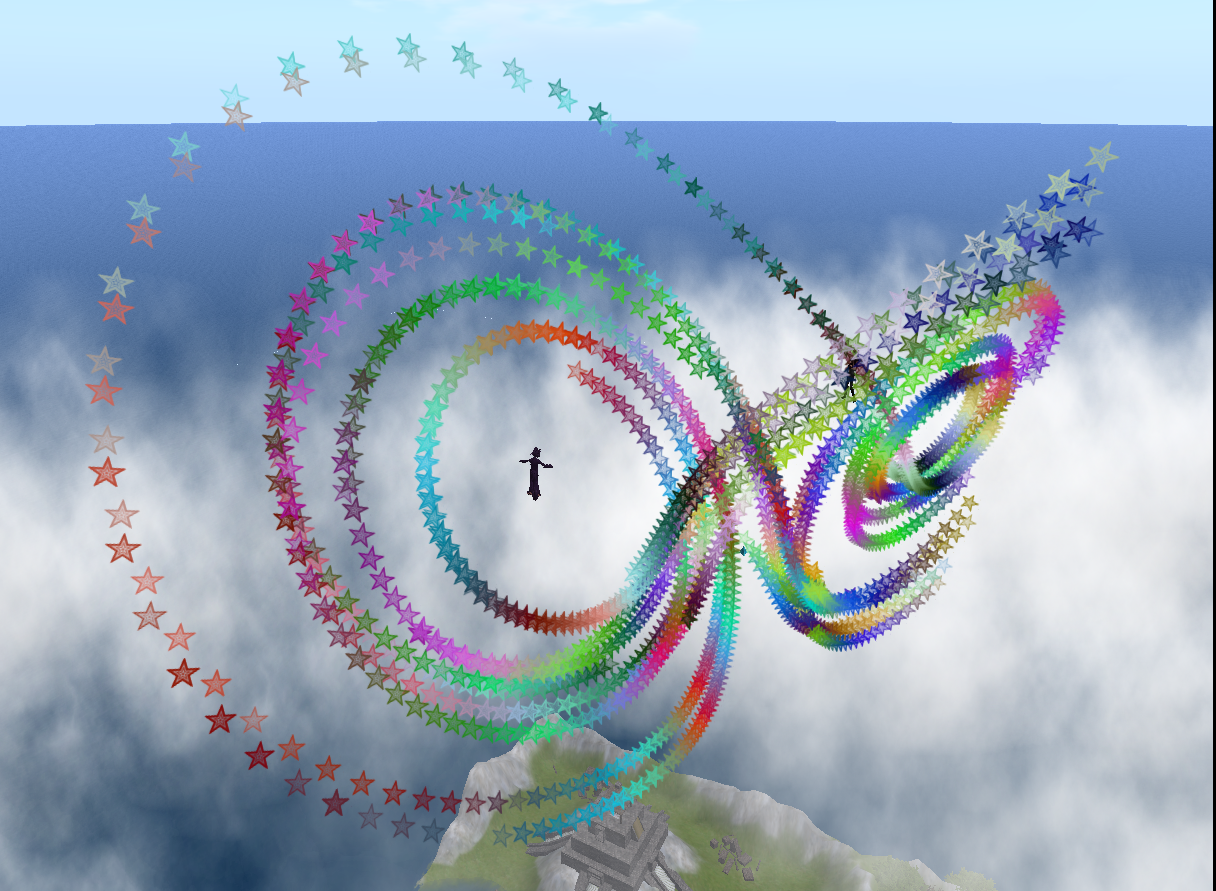
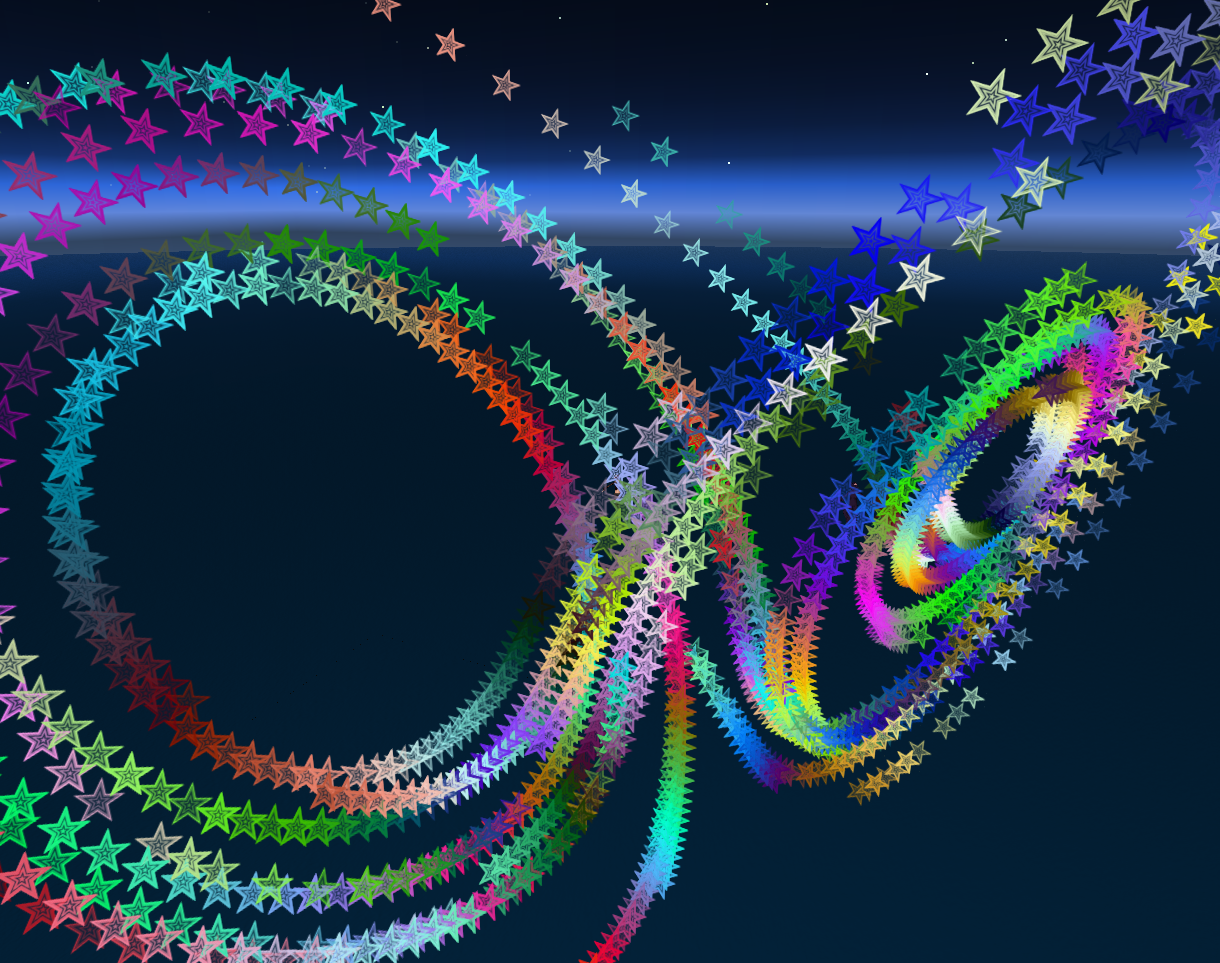
Lorenz Attractor

Visualization of the math non-linear dynamic system called Lorenz attractor.

math lorenz attractor 020
Lorenz attractor, as particle sprites. Scripted by Sleeves Rhode.
math lorenz attractor 015
Lorenz attractor
math lorenz attractor 002
Lorenz attractor
sl lorenz attractor
A plot of the Lorenz attractor as it generates. By Seifert Surface.